pr t hypothesis testing impact|pr in regression model : bespoke 1. The p-value for the intercept and for the effect of speed (i.e. the slope) are for different hypotheses. If you are interested in whether there is a significant . Todo esse conjunto torna a Havan um centro de turismo, lazer e compras, e a loja de departamentos mais completa do Brasil. DEIXE SEU CURRÍCULO PARA NOSSAS .
{plog:ftitle_list}
21 de out. de 2023 · https://www.yaagame.com/2024/01/hentai-heroes.html. October 25, 2023. In this Halloween Parody special, mystery detectives Velma and Daphne .
Here’s how to interpret the values in the Pr (>|t|) column: The p-value for the predictor variable x1 is .0325. Since this value is less than .05, it has a statistically significant relationship with the response variable in the model. The p-value for the predictor variable x2 . Definition 1. p-value (y) = Pr (T (y_rep) >= T (y) | H), where H is a “hypothesis,” a generative probability model, y is the observed data, y_rep are future data under the model, .
This tutorial explains how to interpret the values for Pr(>|t|) in the output of regression models in R, including examples.1. The p-value for the intercept and for the effect of speed (i.e. the slope) are for different hypotheses. If you are interested in whether there is a significant . Hypothesis testing is a way to test a claim or idea about a group or population using sample data. It’s like making an educated guess and then checking if the data supports that guess. A paradigm shift away from null hypothesis significance testing seems in progress. Based on simulations, we illustrate some of the underlying motivations. First, p .
A hypothesis test is a formal statistical test we use to reject or fail to reject some statistical hypothesis. This tutorial explains how to perform the following hypothesis tests in R: .
Tests on individual regression coefficients. Once we have determined that at least one of the regressors is important, a natural next question might be which one(s)? Is the increase in the .
T-Test for Regression. If the regression equation has a slope of zero, then every x x value will give the same y y value and the regression equation would be useless for prediction. We should perform a t-test to see if .For the multiple linear regression model, there are three different hypothesis tests for slopes that one could conduct. They are: Hypothesis test for testing that all of the slope parameters .Hypothesis testing is a statistical method used to determine whether there is a significant difference between two or more sets of data. In the context of Lean Six Sigma, it is primarily used to assess the impact of process changes or .
Step 5: Present your findings. The results of hypothesis testing will be presented in the results and discussion sections of your research paper, dissertation or thesis.. In the results section you should give a brief summary . Importance/Benefits of Hypothesis Testing The most significant benefit of hypothesis testing is it allows you to evaluate the strength of your claim or assumption before implementing it in your data set. Also, hypothesis .
Answer: The key steps involved in conducting hypothesis testing in Six Sigma are: 1) Formulating a clear null and alternative hypothesis based on the problem at hand, 2) Collecting relevant data that is representative of the population under study, 3) Choosing an appropriate statistical test to analyze the data, considering factors such as type .
Introduction. ANOVA (ANalysis Of VAriance) is a statistical test to determine whether two or more population means are different. In other words, it is used to compare two or more groups to see if they are significantly different.. In practice, however, the: Student t-test is used to compare 2 groups;; ANOVA generalizes the t-test beyond 2 groups, so it is used to . I have a doubt regarding the one sample t-test. So as per your book on hypothesis testing with reference to page no 45, you have mentioned the difference between “the sample mean and the hypothesised mean is statistically significant”. So as per my understanding it should be quoted like “the difference between the population mean and the .
Hypothesis testing is a statistical procedure used to test assumptions or hypotheses about a population parameter. It involves formulating a null hypothesis (H0) and an alternative hypothesis (Ha), collecting data, and determining whether the evidence is strong enough to reject the null hypothesis.T-tests use the t-distribution to determine the sampling distribution. For the t-distribution, you need to specify the degrees of freedom, which entirely defines the distribution (i.e., it’s the only parameter). For 1-sample t-tests, the degrees of freedom equal the number of observations minus 1. This dataset has 25 observations.
Hypothesis Testing Rejecting or failing to reject the null hypothesis. Let's return finally to the question of whether we reject or fail to reject the null hypothesis. If our statistical analysis shows that the significance level is below the cut-off value we have set (e.g., either 0.05 or 0.01), we reject the null hypothesis and accept the .The researchers write their hypotheses. These statements apply to the population, so they use the mu (μ) symbol for the population mean parameter.. Null Hypothesis (H 0): The population means of the test scores for the two groups are equal (μ 1 = μ 2).; Alternative Hypothesis (H A): The population means of the test scores for the two groups are unequal (μ 1 ≠ μ 2).
r pr t
The examples on the following pages use the five step hypothesis testing procedure outlined below. This is the same procedure that we used to conduct a hypothesis test for a single mean, single proportion, difference in two means, and difference in two proportions. . 4.1.3 - Impact of Sample Size; 4.2 - Introduction to Confidence Intervals. 4 .Controversies surrounding hypothesis testing. Although hypothesis tests have been the basis of modern science since the middle of the 20th century, they have been plagued by misconceptions from the outset; this has led to what has been described as a crisis in science in the last few years: some journals have gone so far as to ban p-values outright. 6 This is not . Of course, when you perform the hypothesis test, you don’t know which hypothesis is correct. And, the properties of the distribution for the alternative hypothesis are usually unknown. However, use this graph to understand the general nature of these errors and how they are related. T-test definition, formula explanation, and assumptions. The T-test is the test, which allows us to analyze one or two sample means, depending on the type of t-test. Yes, the t-test has several types: One-sample t-test — compare the mean of one group against the specified mean generated from a population. For example, a manufacturer of mobile .
Hypothesis testing with the \(t\)-statistic works exactly the same way as \(z\)-tests did, following the four-step process of. Stating the Hypothesis; Finding the Critical Values; Computing the Test Statistic; Making the Decision. We will work though an example: let’s say that you move to a new city and find a an auto shop to change your oil . 6. Write a null hypothesis. If your research involves statistical hypothesis testing, you will also have to write a null hypothesis. The null hypothesis is the default position that there is no association between the variables. The null hypothesis is written as H 0, while the alternative hypothesis is H 1 or H a.As in simple linear regression, under the null hypothesis t 0 = βˆ j seˆ(βˆ j) ∼ t n−p−1. We reject H 0 if |t 0| > t n−p−1,1−α/2. This is a partial test because βˆ j depends on all of the other predictors x i, i 6= j that are in the model. Thus, this is a test of the contribution of x j given the other predictors in the model.T tests • The z test conditions seldom hold in practice –We don’t often know the population variance –The sample size can be small • We use a T test instead –Assumes the sampling distribution is a t-distribution – ~ Γ(𝜐+1 2) 𝜐𝜋 Γ(𝜐 2) 1+ 𝑥 2 𝜐 2
4. Understand the relation between hypothesis testing, confidence intervals, likelihood and Bayesian methods and their uses for inference purposes. II. The Hypothesis Testing Paradigm and One-Sample Tests A. One-Sample Tests . To motivate the hypothesis testing paradigm we review first two problems. In both cases there is a single sample of data.Using 14.13 as the value of the test statistic for these data, carry out the appropriate test at a 5% level of significance. Show all parts of your test. Answer. In the module on hypothesis testing for means and proportions, we discussed hypothesis testing applications with a dichotomous outcome variable and two independent comparison groups. Introduction to concept It is quite a while since I did any statistics but I think I can answer this one! The bigger the sample size the more likely it is that it represents the true condition of the population. Thus the more reliable is the test. The thing is that the bigger the sample size the more work and potential cost is involved. The trick is to obtain just the right .
PC type Vicat Heating-distortion Temperature Meter sourcing
The hypothesis test is just one part of a research process. There are other pieces that you need to consider. That’s it. That is what a hypothesis test looks like. All hypothesis tests are done with the same six steps. Those general six steps are outlined below. State the random variable and the parameter in words. ## Anova Table (Type II tests) ## ## Response: responses ## Sum Sq Df F value Pr(>F) ## brand 4.3322 1 9.8251 0.004236 ## drops 4.8581 2 5.5089 0.010123 ## Residuals 11.4641 26 The new output switches the columns around and doesn’t show you the mean squares, but gives the most critical parts of the output. In statistics, hypothesis testing is a critical tool. It allows us to make informed decisions about populations based on sample data. Whether you are a researcher trying to prove a scientific point, a marketer analysing A/B test results, or a manufacturer ensuring quality control, hypothesis testing plays a pivotal role.
Hypothesis Testing Framework. The average time for all runners who finished the Cherry Blossom Run in 2006 was 93.29 minutes (93 minutes and about 17 seconds).In null-hypothesis significance testing, the -value [note 1] is the probability of obtaining test results at least as extreme as the result actually observed, under the assumption that the null hypothesis is correct. [2] [3] A very small p-value means that such an extreme observed outcome would be very unlikely under the null hypothesis.Even though reporting p-values of .Let \(D\) denote the data observed. The hypothesis testing is based on the calculation of the probability that such a data set \(D\) and more extreme data can be observed given the hypothesis is true: . The p-value is not the probability that the null hypothesis is true (not \(\Pr(H_0|D)\)), nor is it the probability that the alternative .
Beating Pulp Tester sourcing
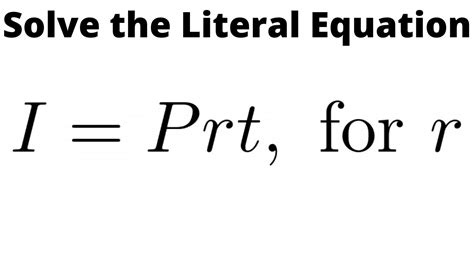
Resultado da 26 de out. de 2021 · About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How YouTube works Test new .
pr t hypothesis testing impact|pr in regression model